
In angle-angle side(AAS) if two angles and the one non-included side of one triangle are congruent to two angles and the non-included side of another triangle, then these two triangles are congruent. In geometry, AAS means angle angle side and is one of the congruence theorems among the 5 different theorems that prove the congruency of two triangles. Whereas the AAS congruence rule states that if two corresponding angles with a non-included side are equal to each other, the two triangles are equal to each other. ASA congruence rule states that if two corresponding angles along with one corresponding side (included in between the angles) are equal to each other, the two triangles are congruent. These two are triangle congruence theorems that help in proving if two triangles are congruent or not.

#Aas geometry plus
We allow this nice of Aas Geometry graphic could possibly be the most trending subject later than we portion it in google plus or facebook. Its submitted by handing out in the best field.
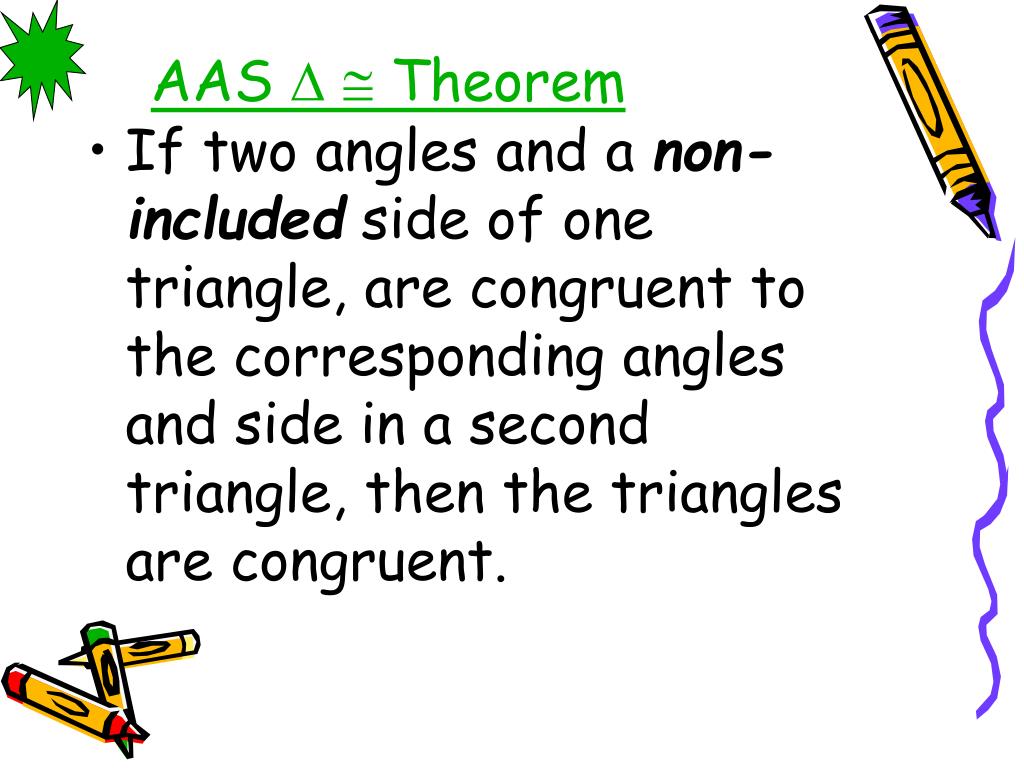
The 4 different triangle congruence rules are: Whereas AAS deals with two angles with a side that is not included in between the two angles of any two given triangles. How Do You Tell if a Triangle is ASA or AAS?īoth the triangle congruence theorems deal with angles and sides but the difference between the two is ASA deals with two angles with a side included in between the angles of any two triangles. The Angle Angle Side Postulate (AAS) states that if two consecutive angles along with a non-included side of one triangle are congruent to the corresponding two consecutive angles and the non-included side of another triangle, then the two triangles are congruent.
#Aas geometry how to
Listed below are a few topics related to the AAS congruence rule, take a look. Angle-Side-Angle (ASA) Rule, Angle-Angle-Side (AAS) Rule, how to use two-column proofs and the rules to prove triangles congruent, geometry, postulates. Therefore, according to the ASA congruence rule, it is proved that ∆ABC ≅ ∆DEF. Some of the worksheets for this concept are Unit 4 triangles part 1 geometry smart packet, Triangle proofs s sas asa aas, Proving triangles congruent. Since we already know that ∠B =∠E and ∠C =∠F, so We also saw if two angles of two triangles are equal then the third angle of both the triangle is equal since the sum of angles is a constant of 180°. In geometry, AAS means angle angle side and is one of the congruence theorems among the 5 different theorems that prove the congruency of two triangles. We know that AB = DE, ∠B =∠E, and ∠C =∠F.
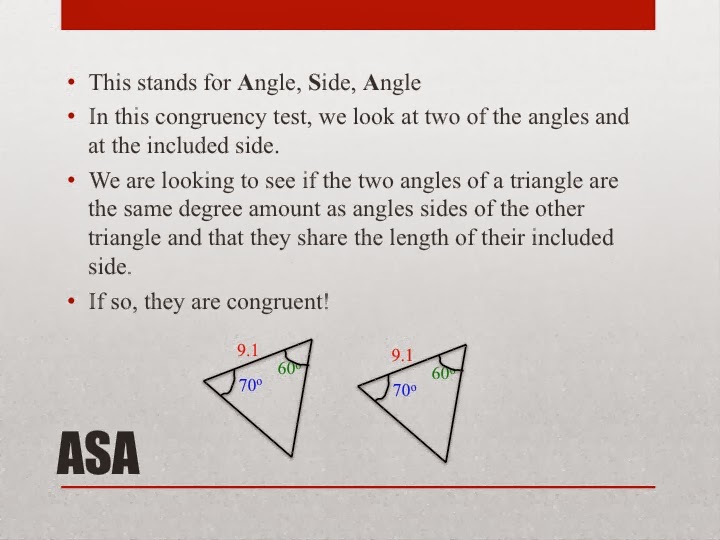
To prove the AAS congruence rule, let us consider the two triangles above ∆ABC and ∆DEF. We should also remember that if two angles of a triangle are equal to two angles of another, then their third angles are automatically equal since the sum of angles in any triangle must be a constant 180° (by the angle sum property).

The AAS congruence rule states that if any two consecutive angles of a triangle along with a non-included side are equal to the corresponding consecutive angles and the non-included side of another triangle, the two triangles are said to be congruent. This works great for guided note- taking or for summarizing and as a study tool.To prove the AAS congruence rule or theorem, we need to first look at the ASA congruence theorem which states that when two angles and the included side (the side between the two angles) of one triangle are (correspondingly) equal to two angles and the included side of another triangle. Perfect for interactive notebooks or as a stand alone graphic organizer. Inside the foldable, there are also 6 examples where students will tell which postulate, if either, can prove the triangles are congruent. This foldable provides students with written postulates/theorems, a diagram, and if-then congruence statements for the Angle-Side-Angle Postulate and Angle-Angle-Side Theorem.


 0 kommentar(er)
0 kommentar(er)
